0 Comments
0 Shares
0 Reviews

Directory
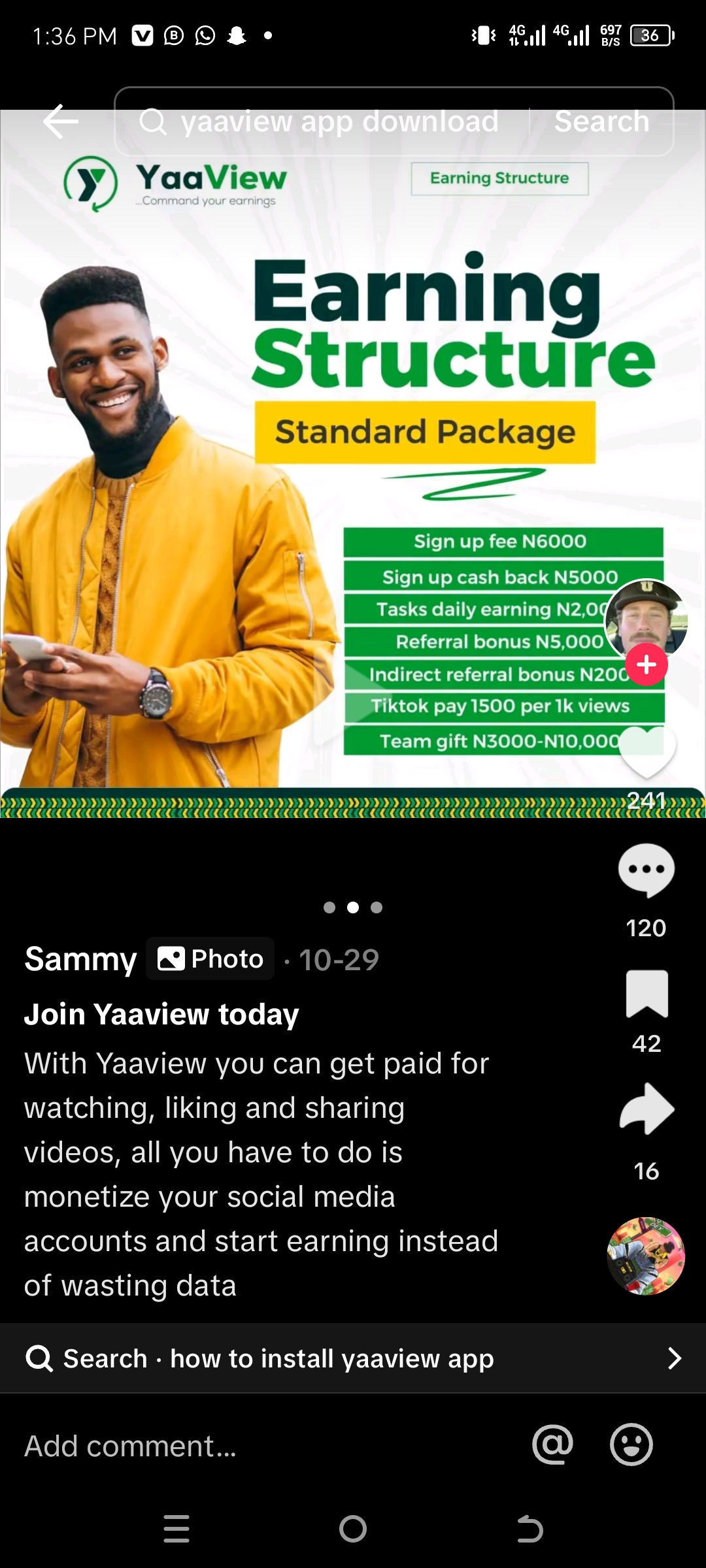
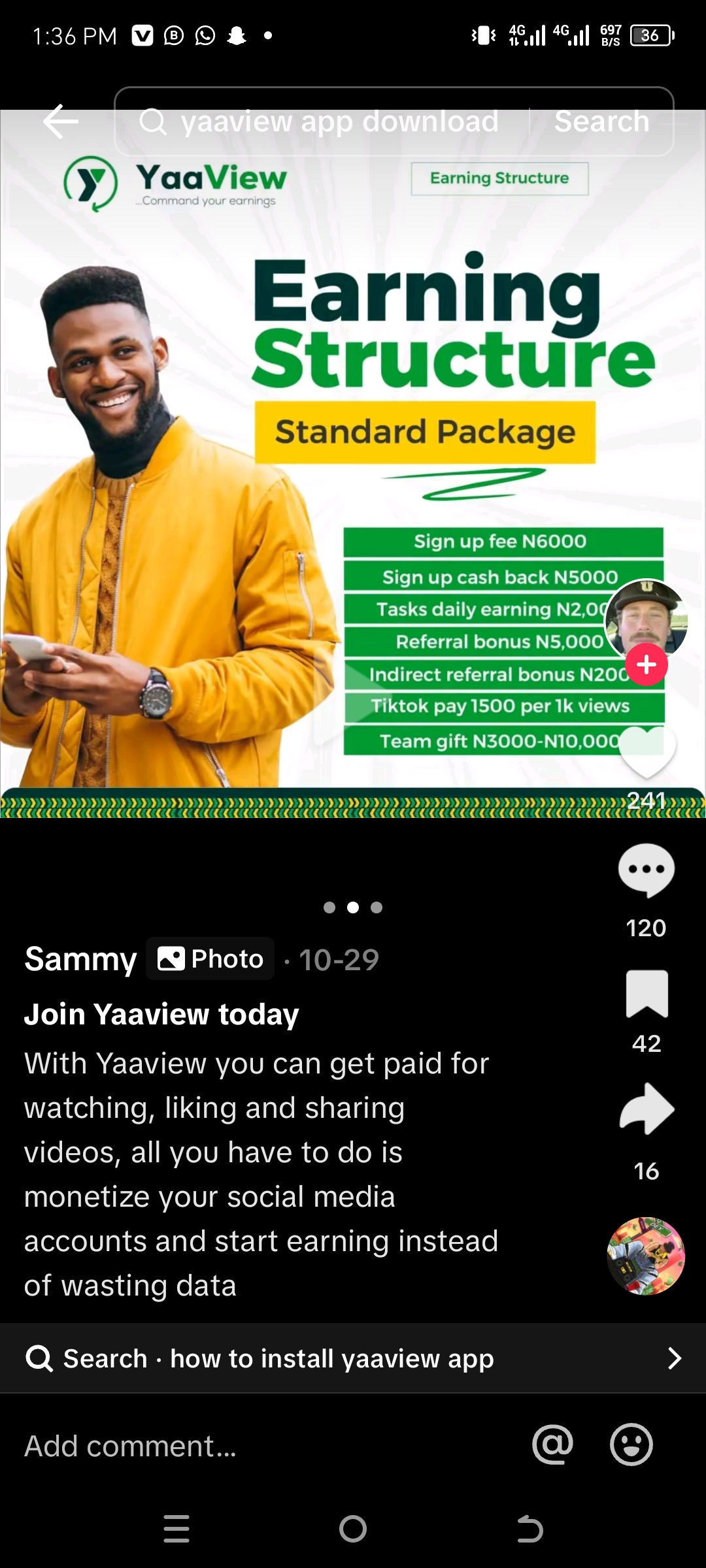
Genuine Profiles ONLY! Connect & Create. Be Rewarded. With Danloader, You Get to Find only genuine people, create genuine connections, and make genuine friends. You also earn rewards.
-
Please log in to like, share and comment!
-
-
-
-
-
-
Life is like a big merry go round, taking us back to the spot we've been. Please do good because life may recycle people you've hurt, and trust me, you won't be able to turn out the hands of time.Life is like a big merry go round, taking us back to the spot we've been. Please do good because life may recycle people you've hurt, and trust me, you won't be able to turn out the hands of time.0 Comments 0 Shares 0 Reviews
-
"No one in this world can ever love you more than your parents.""No one in this world can ever love you more than your parents."0 Comments 0 Shares 0 Reviews
-
You could give some people a drop of water and they'd still appreciate you. You could give other people the entire ocean and they'd still take you for granted.You could give some people a drop of water and they'd still appreciate you. You could give other people the entire ocean and they'd still take you for granted.0 Comments 0 Shares 0 Reviews
-
Sponsored
Sponsored
Sponsored
Sponsored
Sponsored